Mean
Subject: Business Statistics

Overview
The average or midpoint for a given set of data values is the measure of central tendency. There are generally three different types of central tendency measures. Mean, median, and mode are the three. The most popular and widely used way to determine the average for a given set of data values is to utilize the mean.
Measure Of Central Tendency
The mean, the mode, and the median are the three primary forms of Measure of Central Tendency. The average or the midpoint for a given set of data values is a measure of central tendency.
Mean:
The sum of the data values divided by the total number of data values in the set is the definition of the mean of a set of data values. For sample data, it is indicated as \(\overline(x)\) and for population data µ, it is presented as:
Mean= \(\frac{Total \;sum \;of \;data}{\text{Number of data}}\)
Mean= \(\frac{∑x}{n}\)
Thus, the population means μ = ∑x/n.
Example 1:
Suppose we have the following observations:
7, 15,30, 10, 83, 79 and 42
These are seven observations. Symbolically, the arithmetic mean is also called simply
mean is:
=\(\frac{Total\; sum\; of\; data}{Number\;of\;data}\)
=\(\frac{10+15+30+7+42+79+83}{7}\)
=\(\frac{266}{7}\)
=38
The arithmetic mean is defined by the basic formula given above, which is applied to ungrouped data without the use of weights or frequencies.
Weighted Mean:
The average determined in relation to the frequency of the data values.
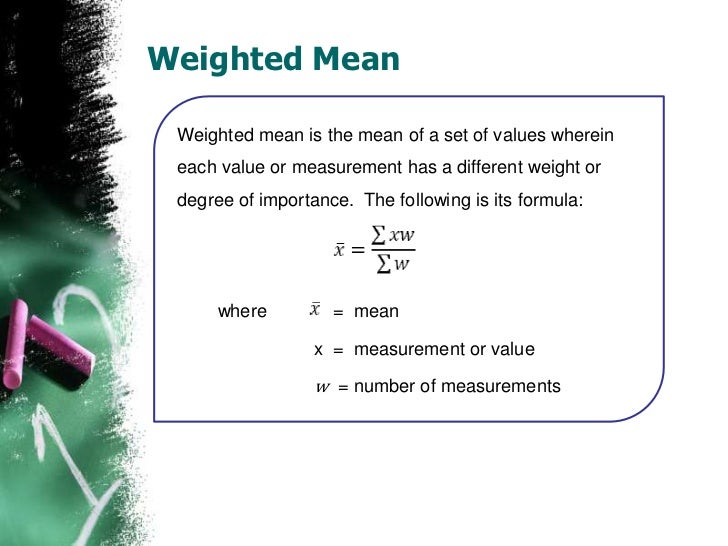
Example 2:
You take three 100 marks exams for the statistics class and score 80, 80 and 85. The last exam is much easier than the first two, so your teacher assigned some weights to the subjects. The weights for the three exams are:
- Exam 1: 40 % of your grade. (Note: 40% as a decimal is .4.)
- Exam 2: 40 % of your grade.
- Exam 3: 20 % of your grade
| Exam | Weights | Marks | w.m |
| Exam-1 | .4 | 80 | 32 |
| Exam-2 | .4 | 80 | 32 |
| Exam-3 | .2 | 85 | 19 |
As,we Know,
=\(\frac{83}{1}\)
=83
Arithmetic mean for Grouped Data:
If the number of items is large then the data is grouped. In this case, Mean is calculated by using the following three methods:
- \(Direct \: method, \overline{X} = \frac{\sum fm}{n}\), where m is the mid-value of class intervals.
- \(Short \: cut \: method,\overline{X} = A + \frac{\sum fd}{n} \), where d= X - A and A is assumed mean.
- \(Step \: deviation \: method, \overline{X} = A + \frac{\sum fd'}{n}\) \( \times h \), where d' = \(\frac{d}{h}\) and h is the length of class interval.
Example 3:
The following table gives the marks of 58 students in Business Statistics. Calculate the average marks based on the table below..
| Marks | No.Of Students |
| 0-10 | 4 |
|
10-20 |
8 |
| 20-30 | 11 |
| 30-40 | 15 |
| 40-50 | 12 |
| 50-60 | 6 |
| 60-70 | 2 |
| total | 58 |
Solution:
Calculation of arithmetic mean by direct method:
| Marks | Mid-point | Frequency | fm |
| 0-10 | 5 | 4 | 20 |
| 10-20 | 15 | 8 | 120 |
| 20-30 | 25 | 11 | 275 |
| 30-40 | 35 | 15 | 525 |
| 40-50 | 45 | 12 | 540 |
| 50-60 | 55 | 6 | 330 |
| 60-70 | 65 | 2 | 130 |
| total | 58 | 1940 |
\(\overline{X} = \frac{\sum fm}{n}\), where m is the mid-value of class intervals.
=\(\frac{1940}{58}\)
=33.45=33(approximately)
Shortcut Method:
The idea of an arbitrary mean is used in the case of the shortcut technique. The following is the formula for calculating the arithmetic mean using the shortcut method:
\(Short \: cut \: method,\overline{X} = A + \frac{\sum fd}{n} \), where d= X - A and A is assumed mean.
- f = frequency
- d = deviation from the arbitrary or assumed mean
The direct method would not be appropriate to employ when the quantities are very large and/or in fractions. The shortcut approach is better to the direct approach in certain circumstances. This is due to a significant reduction in computation labor when using the shortcut approach, especially when calculating the product of values and their respective frequencies. While any value may be used as the assumed mean, it would be appropriate to avoid extreme values, that is, too low or high, in order to make calculations simpler. It is best to select a number that is near to the arithmetic mean.
Example 4:
For the above example 3, we will now use shortcut method. Let us assume the mean to be 35.
So, a=35
| Marks | Mid-point | f | d=m-a | fd |
| 0-10 | 5 | 4 | -30 | -120 |
| 10-20 | 15 | 8 | -20 | -160 |
| 20-30 | 25 | 11 | -10 | -110 |
| 30-40 | 35 | 15 | 0 | 0 |
| 40-50 | 45 | 12 | 10 | 120 |
| 50-60 | 55 | 6 | 20 | 120 |
| 60-70 | 65 | 2 | 30 | 60 |
| total | -90 |
Short cut method: Overline{X} = A + \frac{\sum fd}{n} \), where d= X - A and A is assumed mean.
= 35 - 1.55 = 33.45 or 33 marks approximately.
For the same scenario, try the step deviation approach.
Characteristics of Mean:
- The deviation's algebraic sum is equal to zero since the sums of the deviations on either side of the mean are identical.
- A data set can only have one mean.
- Any time a data value changes, the arithmetic mean also changes.
- The values' order is not required.
Reference:
Kunda, Surinder. An Introduction to business statistics. n.d.
Things to remember
- The average or the midpoint for a given set of data values is a measure of central tendency.
- The mean, the mode, and the median are the three primary forms of Measure of Central Tendency.
- The sum of the data values divided by the total number of data values in the set is the definition of the mean of a set of data values. For sample data, it is indicated as (overline(x)) and for population data µ, it is presented as:
- Mean= \(\frac{Total sum of data}{Number of data}\)
- The weighted mean is the mean that has been calculated with reference to the frequency of the data value.
- When there are many elements, the data is grouped. The three techniques listed below are used to determine Mean in this situation:
- \(Direct \: method, \overline{X} = \frac{\sum fm}{n}\), where m is the mid-value of class intervals.
- \(Short \: cut \: method,\overline{X} = A + \frac{\sum fd}{n} \), where d= X - A and A is assumed mean.
- \(Step \: deviation \: method, \overline{X} = A + \frac{\sum fd'}{n}\) \( \times h \), where d' = \(\frac{d}{h}\) and h is the length of class interval.
- Characteristics of Mean:
- The deviation's algebraic sum is equal to zero since the sums of the deviations on either side of the mean are identical.
- A data set can only have one mean.
- Any time a data value changes, the arithmetic mean also changes.
- The values' order is not required.
© 2021 Saralmind. All Rights Reserved.

 Login with google
Login with google